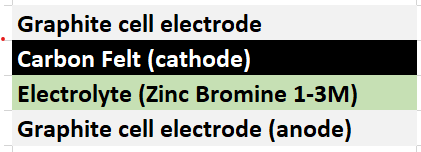
Static Zn-Br batteries have gained popularity during the past 4 years due to their apparent simplicity and their theoretical ability to last thousands of cycles without deterioration at a very low cost (although this is often extrapolated from Zn-Br flow batteries). The particular configuration that has become popular among some researchers and DIY enthusiasts is the one shown below, where the Zn-Br battery is assembled with the cathode at the bottom of the battery and either a graphite or Zinc metal anode at the top, with the electrolyte in between both of them.

In this configuration for the battery, elemental Bromine (or sequestered bromine if any sequestering agents are used) will tend to accumulate at the bottom. This happens because Bromine is both denser than water and attracted to the carbon felt electrode. As bromine is insoluble in water and prefers to interact with the felt. The Zinc is then deposited at the top electrode and the cell only appears to be limited by the self-discharge of the process, caused by Bromine diffusion from cathode to anode.
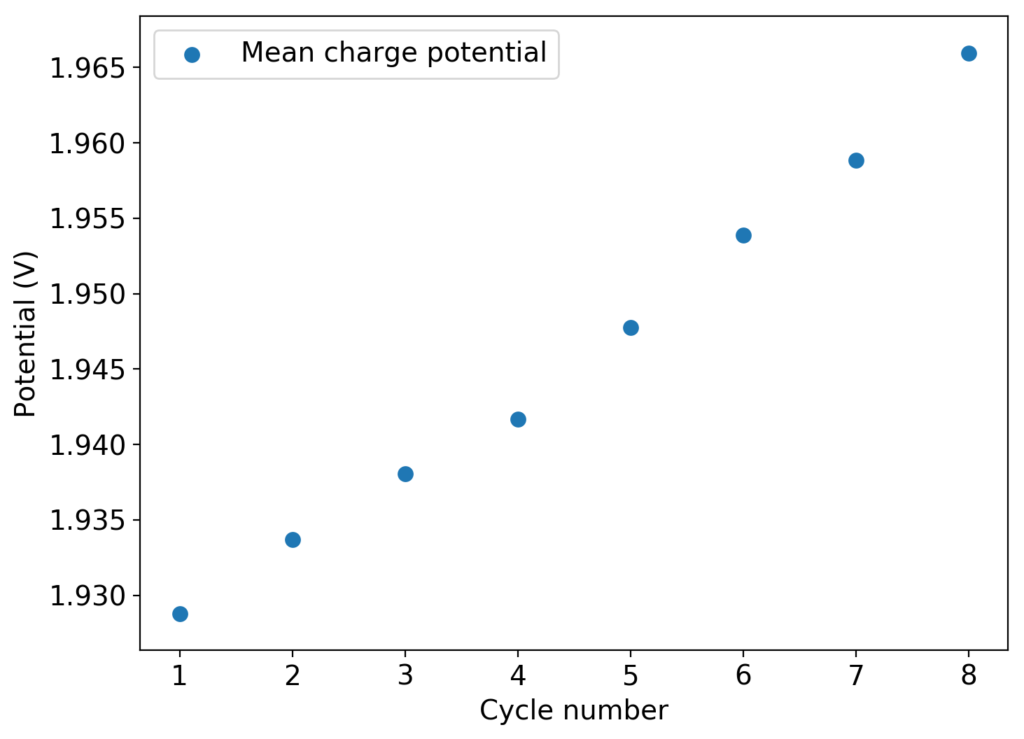
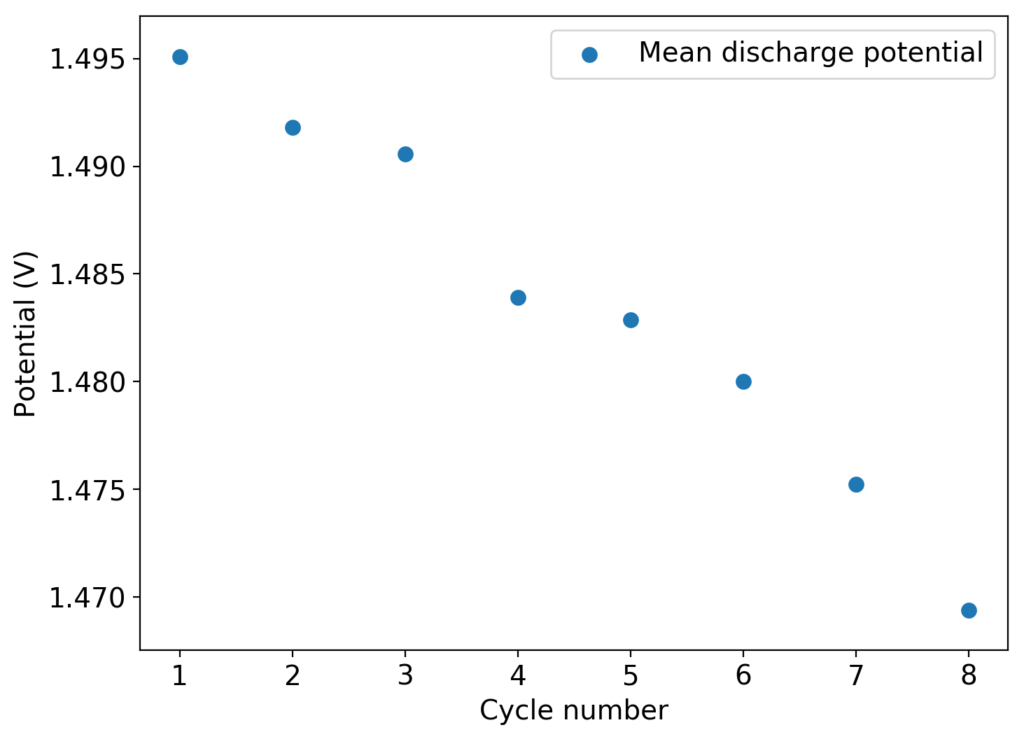
However, experimentally – as you can see extensively in my work in this blog – at practical current densities (>15 mA/cm2), even in devices with anode/cathode distances of only 2-3mm, there is a substantial evolution of hydrogen in the anode due to the overpotential required to overcome the internal resistance of the device. This is true for ZnBr2 concentrations of 1.5-3M. This means that a lot of hydrogen is produced, which is then either trapped against the anode – reducing its surface – or then leaves the device at the expense of making the solution more alkaline, both processes which inevitably kill the device as a function of time. Trying to increase the conductivity further also leads to other, worse problems, such as robust Zinc dendrite formation.
To deal with the above means that you either need to periodically replace the electrolyte or treat it in some manner. This might not be economical if additives like sequestering agents are used but it is definitely not desirable as you will have to deal with a lot of left-over bromine containing solution. A potential solution might be to replenish the battery by adding HBr – in an analogous way to how you replenish the sulfuric acid in a lead acid battery, but this solution is not likely to be very practical due to the makeup of this battery. This is because adding excess HBr makes the hydrogen evolution problem much worse, so careful titration of the solution with the HBr is required in order to arrive at just the right pH, very impractical for users.
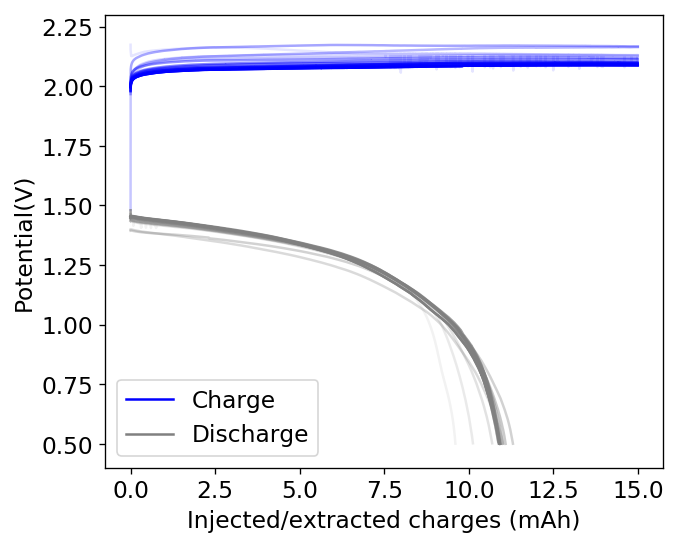
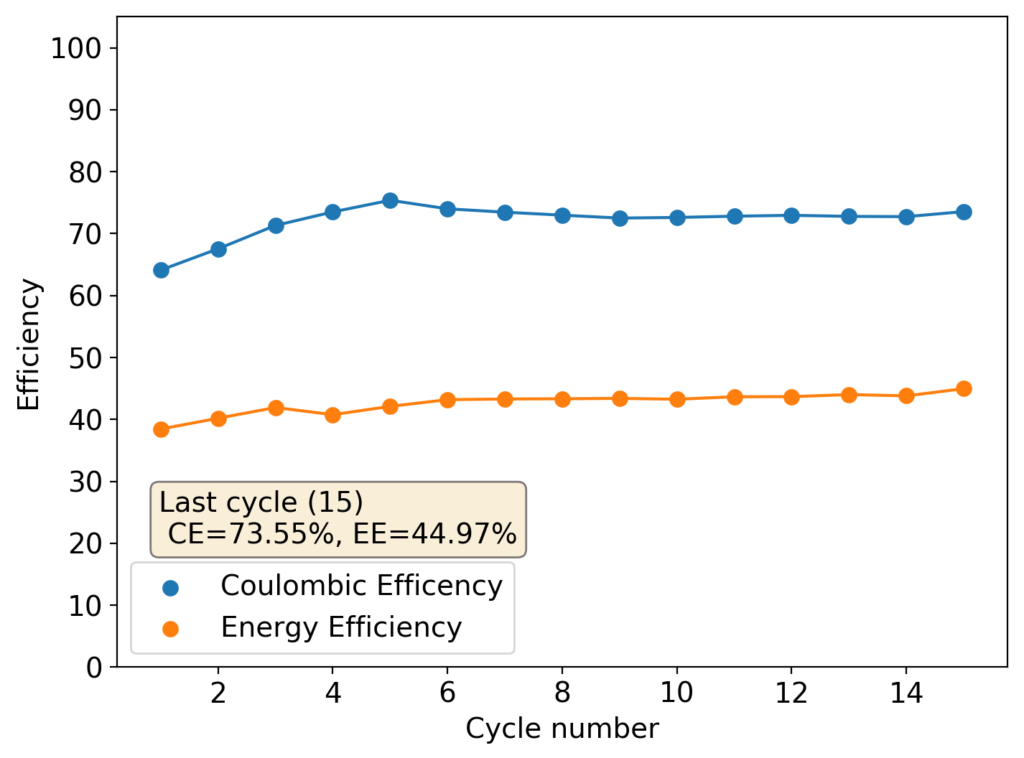
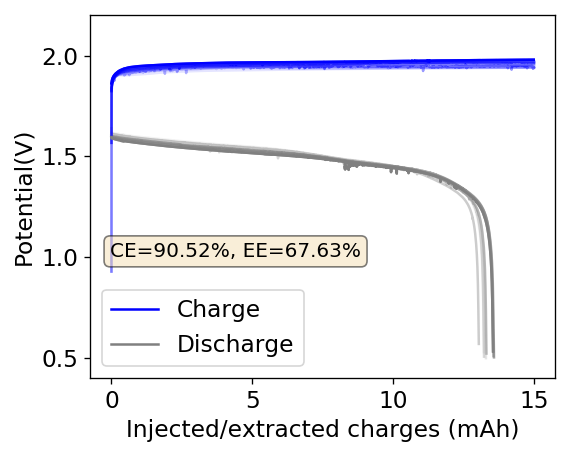
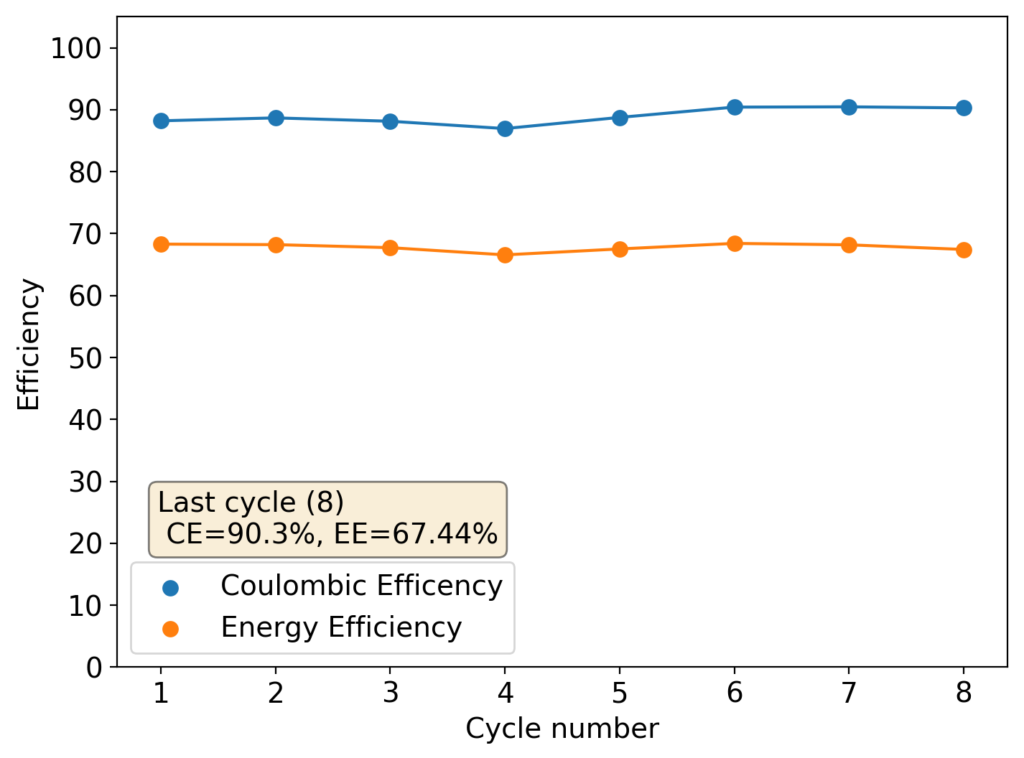
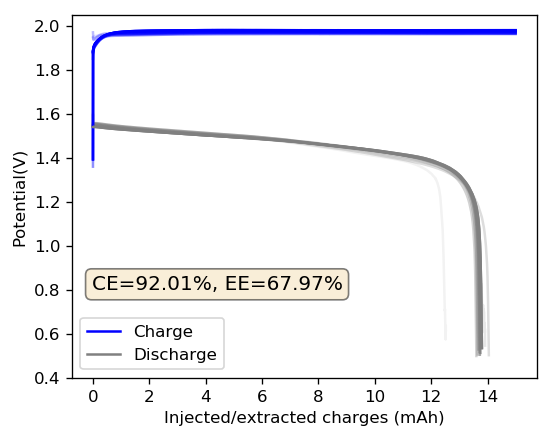
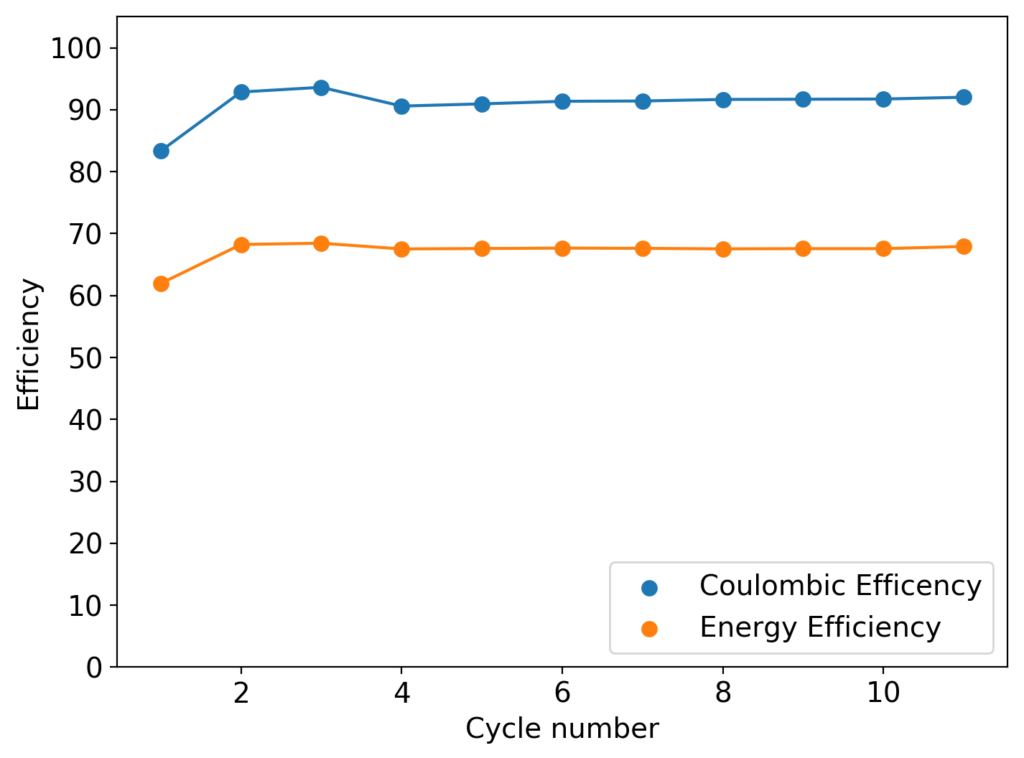
A more permanent solution is to use an inverted architecture, where the cathode is placed at the top and the anode at the bottom. Any hydrogen gas created then reacts with the solution and cathode, regenerating the electrolyte in the process. This sadly decreases the Coulombic and Energy efficiency value of the device, because Bromine diffusion is substantially aided by its tendency to sink into a water solution. Experimentally the CE drops from around 90% to 70% and the EE from 70% to 50% at the same current densities (see here). However this is likely where we need to start if we really want a Zn-Br architecture that can be used for a long amount of cycles in practice.
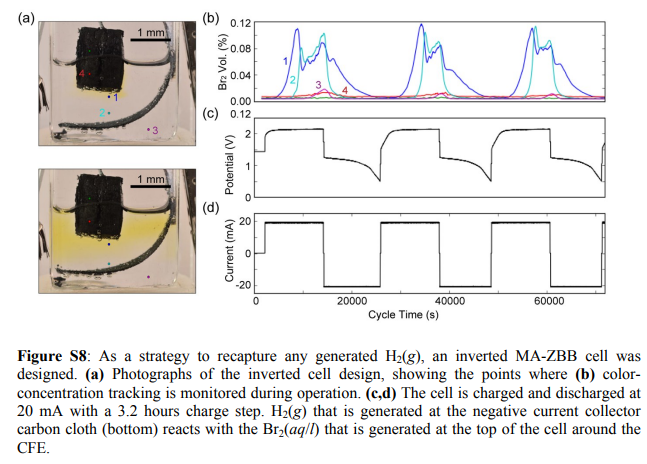
In order to make this architecture viable we would need to increase the affinity of the Br2 for the felt and prevent it from sinking into the water solution, a very challenging proposition but one we can work towards if we start from a solid base. Soaking the felt in an organic phase that is conductive enough is the first things I am going to try to get to this goal.